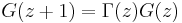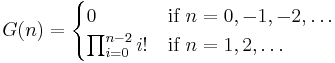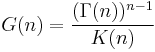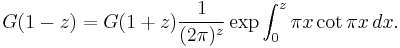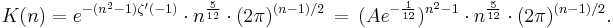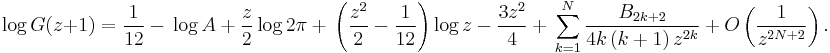Barnes G-function

In mathematics, the Barnes G-function G(z) is a function that is an extension of superfactorials to the complex numbers. It is related to the Gamma function, the K-function and the Glaisher–Kinkelin constant, and was named after mathematician Ernest William Barnes.[1] Up to elementary factors, it is a special case of the double gamma function.

Formally, the Barnes G-function is defined (in the form of a Weierstrass product) as

G(z%2B1)=(2\pi)^{z/2} \exp(-(z(z%2B1)%2B\gamma z^2)/2)\ \times\ \prod_{n=1}^\infty \left[\left(1%2B\frac{z}{n}\right)^n \exp(-z%2Bz^2/(2n))\right],

where γ is the Euler–Mascheroni constant, exp(x) = ex, and ∏ is capital pi notation.

Contents

Difference equation, functional equation and special values

The Barnes G-function satisfies the difference equation

G(z%2B1)=\Gamma(z)G(z)

with normalisation G(1) = 1. The difference equation implies that G takes the following values at integer arguments:

G(n)=\begin{cases} 0&\text{if }n=0,-1,-2,\dots\\ \prod_{i=0}^{n-2} i!&\text{if }n=1,2,\dots\end{cases}

and thus

G(n)=\frac{(\Gamma(n))^{n-1}}{K(n)}

where Γ denotes the Gamma function and K denotes the K-function. The difference equation uniquely defines the G function if the convexity condition: \frac{d^3}{dx^3}G(x)\geq 0 is added.[2]

The difference equation for the G function and the functional equation for the Gamma function yield the following functional equation for the G function, originally proved by Hermann Kinkelin:

G(1-z) = G(1%2Bz)\frac{ 1}{(2\pi)^z} \exp \int_0^z \pi x \cot \pi x \, dx.

Multiplication formula

Like the Gamma function, the G-function also has a multiplication formula[3]:

G(nz)= K(n) n^{n^{2}z^{2}/2-nz} (2\pi)^{-\frac{n^2-n}{2}z}\prod_{i=0}^{n-1}\prod_{j=0}^{n-1}G\left(z%2B\frac{i%2Bj}{n}\right)

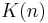where K(n) is a constant given by:

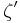K(n)= e^{-(n^2-1)\zeta^\prime(-1)} \cdot n^{\frac{5}{12}}\cdot(2\pi)^{(n-1)/2}\,=\, (Ae^{-\frac{1}{12}})^{n^2-1}\cdot n^{\frac{5}{12}}\cdot (2\pi)^{(n-1)/2}.

Here \zeta^\prime is the derivative of the Riemann zeta function and A is the Glaisher–Kinkelin constant.

Asymptotic expansion

The logarithm of G(z + 1) has the following asymptotic expansion, as established by Barnes:

\log G(z%2B1)=\frac{1}{12}~-~\log A~%2B~\frac{z}{2}\log 2\pi~%2B~\left(\frac{z^2}{2} -\frac{1}{12}\right)\log z~-~\frac{3z^2}{4}~%2B~ \sum_{k=1}^{N}\frac{B_{2k %2B 2}}{4k\left(k %2B 1\right)z^{2k}}~%2B~O\left(\frac{1}{z^{2N %2B 2}}\right).

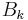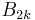Here the B_{k} are the Bernoulli numbers and A is the Glaisher–Kinkelin constant. (Note that somewhat confusingly at the time of Barnes [4] the Bernoulli number B_{2k} would have been written as 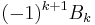(-1)^{k%2B1} B_k , but this convention is no longer current.) This expansion is valid for 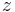z in any sector not containing the negative real axis with |z| large.

References

  1. ^ E.W.Barnes, "The theory of the G-function", Quarterly Journ. Pure and Appl. Math. 31 (1900), 264–314.
  2. ^ M. F. Vignéras, L'équation fonctionelle de la fonction zêta de Selberg du groupe mudulaire SL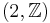(2,\mathbb{Z}), Astérisque 61, 235–249 (1979).
  3. ^ I. Vardi, Determinants of Laplacians and multiple gamma functions, SIAM J. Math. Anal. 19, 493–507 (1988).
  4. ^ E.T.Whittaker and G.N.Watson, "A course of modern analysis", CUP.